|
ADVANCE/DECLINE RATIO
Overview
The Advance/Decline Ratio ("A/D Ratio") shows the ratio of advancing issues to declining
issues. It is calculated by dividing the number of advancing issues by the number of
declining issues.
Interpretation
The A/D Ratio is similar to the Advancing-Declining Issues in
that it displays market breadth. But, where the Advancing-Declining Issues subtracts the
advancing/declining values, the A/D Ratio divides the values. The advantage of the Ratio
is that it remains constant regardless of the number of issues that are traded on the New
York Stock Exchange (which has steadily increased).
A moving average of the A/D Ratio is often used as an overbought/oversold indicator.
The higher the value, the more "excessive" the rally and the more likely a correction.
Likewise, low readings imply an oversold market and suggest a technical rally.
Keep in mind, however, that markets that appear to be extremely overbought or oversold
may stay that way for some time. When
investing using overbought and oversold indicators,
it is wise to wait for the prices to confirm your belief that a change is due before
placing your trades.
Day-to-day fluctuations of the Advance/Decline Ratio are often eliminated by smoothing
the ratio with a moving average.
Example
The following chart shows the S&P 500 and a
15-day moving average of the A/D Ratio.
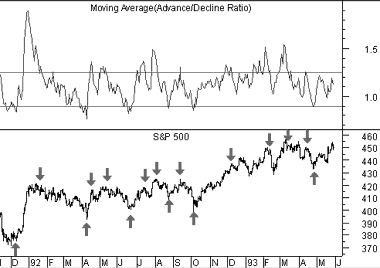 You can see that prices usually declined after
entering the overbought level above 1.25 ("sell" arrows) and that they usually rallied
after entering the oversold level below 0.90 ("buy" arrows).
You can see that prices usually declined after
entering the overbought level above 1.25 ("sell" arrows) and that they usually rallied
after entering the oversold level below 0.90 ("buy" arrows).
Calculation
The A/D Ratio is calculated by dividing the number of stocks that advanced in price for the
day by the number of stocks that declined.
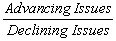
Table 3 shows the calculation of the A/D Ratio.
|
Table 3 |
| Date |
Advancing |
Declining |
A/D Ratio |
| 02/15/94 |
1198 |
882 |
1.3583 |
| 02/16/94 |
1183 |
965 |
1.2259 |
| 02/17/94 |
882 |
1251 |
0.7050 |
| 02/18/94 |
706 |
1411 |
0.5004 |
| 02/22/94 |
1139 |
1003 |
1.1356 |
|

